Continuity From Graph Practice With Answers

SKETCH THE GRAPH AND VERIFY THE CONTINUITY OF THE FUNCTION

About "Sketch the Graph and Verify the Continuity of the Function"

Sketch the Graph and Verify the Continuity of the Function :

Here we are going to see some example problems to understand the concept of sketching the graph and verifying the continuity of the function.

Sketch the Graph and Verify the Continuity of the Function - Practice questions

Question 1 :

Let f(x)

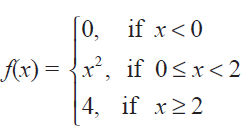
Graph the function. Show that f(x) continuous on (- ∞, ∞).

Solution :

There are three partitions in the piecewise function.

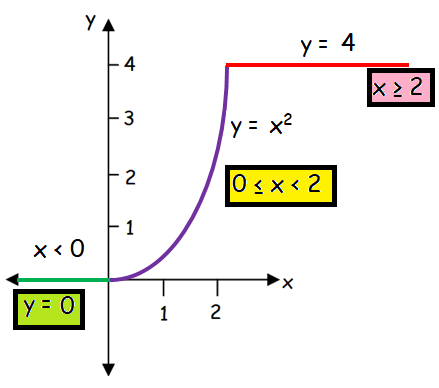
To check the continuity at the point 0, we should prove the following.

lim _{x-> 0-} f(x)  =  lim _x->0+ f(x)  =  lim _x->0 f(0)

lim_{x-> 0-} f(x)  =  0   ----(1)

lim_x->0+ f(x)  =  0   ----(2)

f(0)  =  0   ----(3)

(1)  =  (2)  =  (3)

Hence the function is continuous at x = 0.

Now let us check the continuity at the point 2.

lim_{x-> 2-} f(x)  =  lim_x->2+ f(x)  =  lim _x->2 f(0)

lim_{x-> 2-} f(x)  =  2²  =  4   ----(1)

lim_x->2+ f(x)  =  4   ----(2)

f(2)  =  4   ----(3)

(1)  =  (2)  =  (3)

Hence the function is continuous at x = 2.

So the given piecewise function is continuous on (- ∞, ∞).

Question 2 :

If f and g are continuous functions with f(3) = 5 and lim x->3 [2 f(x) - g(x)]  =  4, find g(3).

Solution :

lim _x->3 [2 f(x) - g(x)]  =  4

Let us apply 3 in the function instead of x.

[2 f(3) - g(3)]  =  4

2(5) - g(3)  =  4

10 - g(3)  =  4

g(3)  =  10 - 4

g(3)  =  6

Hence the value of g(3) is 6.

Question 3 :

Find the points at which f is discontinuous. At which of these points f is continuous from the right, from the left, or neither? Sketch the graph of f.

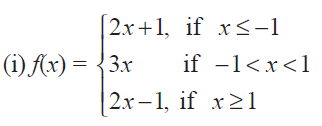
Solution :

First let us check the continuity at the point x  =  -1

lim _{x-> -1-} f(x)  =  lim_{x-> -1-} 2x + 1

By applying the limit, we get

  =  2(-1) + 1

  =  -2 + 1

  =  -1 -----(1)

lim_{x-> -1+} f(x)  =  lim_{x-> -1+} 3x

By applying the limit, we get

  =  3(-1)

  =  -3 -----(2)

lim _{x-> -1-}  f(x) ≠   lim_{x-> -1+}

So, the function is not continuous at x = -1.

Now let us check the continuity at the point x  =  1

lim_{x-> 1-} f(x)  =  lim_{x-> 1-} 3x

By applying the limit, we get

  =  3(1)

  =  3 -----(1)

lim_{x-> -1+} f(x)  =  lim_{x-> -1+} 2x - 1

By applying the limit, we get

  =  2(1) - 1

  =  1 -----(2)

lim _{x-> 1-}  f(x) ≠   lim_{x-> 1+}

So, the function is not continuous at x = 1.

To find at which of these points f is continuous from the right, from the left, or neither, we have to draw the number line.

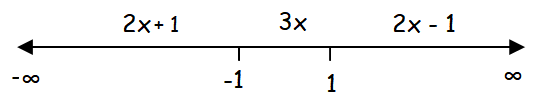
let x₀∈ (- ∞, -1]

lim _{x-> x0} f(x)  =  lim_{x-> x0} 2x + 1

Applying the limit, we get

  =  2x₀ + 1  ------(1)

f(x₀)  =  2x₀ + 1  ------(2)

(1)  =  (2)

It is continuous in  (- ∞, -1].

letx₀∈ (-1 , -1)

lim_{x-> x0} f(x)  =  lim_{x-> x0} 3x

Applying the limit, we get

  =  3x₀  ------(1)

f(x₀)  =  3x₀   ------(2)

(1)  =  (2)

It is continuous in  (-1 , 1).

letx₀∈ [1 , ∞ )

lim_{x-> x0} f(x)  =  lim_{x-> x0} 2x - 1

Applying the limit, we get

  =  2x₀ - 1  ------(1)

f(x₀)  =2x ₀ - 1   ------(2)

(1)  =  (2)

It is continuous in [1 , ∞ ).

Graph of f(x) = 2x + 1 :

x = -1 f(-1)  =  -1

x = -2 f(-2)  =  -3

x = -3 f(-3)  =  -5

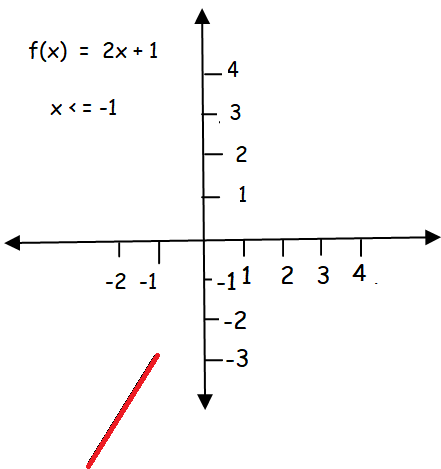
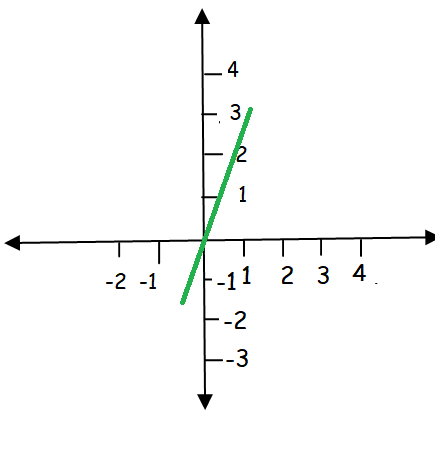
Graph of f(x) = 3x :

-1 < x < 1

x = -0.5 f(-0.5)  =  -1.5

x = -0.7 f(-0.7)  =  -2.1

x = 0.5 f(0.5)  =  1.5

Graph of f(x) = 2x - 1:

x > = 1

x = 1 f(1)  =  1

x = 2 f(2)  =  3

x = 3 f(3)  =  5

After having gone through the stuff given above, we hope that the students would have understood, " Sketch the Graph and Verify the Continuity of the Function"

Apart from the stuff given in " Sketch the Graph and Verify the Continuity of the Function" , if you need any other stuff in math, please use our google custom search here.

Kindly mail your feedback tov4formath@gmail.com

We always appreciate your feedback.

© All rights reserved. onlinemath4all.com

whitfordamiled.blogspot.com

Source: https://www.onlinemath4all.com/sketch-the-graph-and-verify-the-continuity-of-the-function.html

Share this post